Introduction
The immense importance of Einstein Field Equations (“EFE”) warrants a thorough understanding of every single element contained within them. Einstein’s equation is another name for the Einstein Field Equation. Ten equations make up this set which is derived from the General Theory of Relativity of Albert Einstein.
This tutorial aims to present a tight, clear, and simple logic of EFE that is also comprehensive so that any general mathematician can understand EFE by simply reading this tutorial. In this tutorial, we will describe Einstein Field Equations, metric tensor, Einstein tensor, properties of Einstein tensor, the cosmological constant, and Practical Application of Einstein Field Equations.
The Einstein Field Equations
By relativity theory, Einstein’s field equations correspond to spacetime geometrical points. It is determined by the separation of matter within it. Einstein proposed the equation in 1915. It took the shape of a tensor equation by which spacetime curvature is linked to energy, momentum, and stress. The tensor equation is the result of ten different equations being combined. It characterizes gravity as an outcome of the curvature of space-time caused by both mass and energy. The curvature of time and space at a specific point in time and space determines it. At that point, it is also associated with momentum and energy. Maxwell’s equations relate electromagnetic fields to the distribution of charges and currents, just as Einstein’s field equations relate mass-energy, momentum, and stress to spacetime geometry. These equations’ solutions are components of the metric tensor, which specifies the space-time geometry. The geometric equations can then be used to calculate the pathways of these particles.
Einstein field equation can be written as a series of non-partial differential equations due to the relationship between the metric tensor and the Einstein tensor.
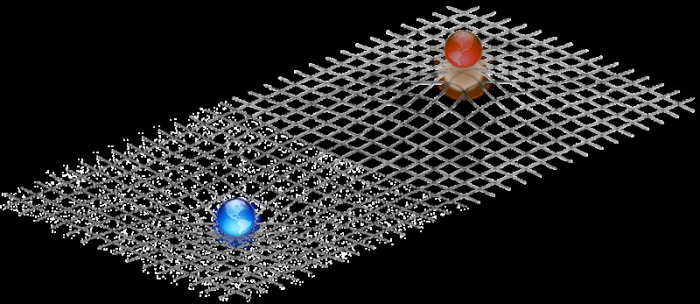
Tokamac, One-sided spacetime curvatures, CC BY-SA 4.0
2D rubber-sheet model of a curved spacetime according to the Einstein field equations. Positive mass (blue) and negative mass (red) share the same single “side” of a 4D hypersurface.
Explore our latest online courses and learn new skills at your own pace. Enroll and become a certified expert to boost your career.
Einstein Field Equations are
Gμv=8πGTμVGμv=8πGTμV
G = Newton constant
Gμv=Rμv−12RgμvGμv=Rμv−12Rgμv is the Einstein tensor is a measure of space-time curvature.
TμvTμv is the energy-momentum tensor.
gμvgμv is the metric tensor.
RμvRμv Ricci tensor
Metric tensor
In the case of curved space-time, the metric tensor is essentially a replacement for the Kronecker delta.
In special relativity the invariant is −
dτ2=c2dt2−dx2−dy2−dz2=nαβdxαdxβdτ2=c2dt2−dx2−dy2−dz2=nαβdxαdxβ
The principle of relativity states that there is always a falling coordinate frame ξαξα in which dξαdτ2dξαdτ2 vanishes.. In another coordinate frame xμxμ, the invariant becomes
dτ2=nαβ∂ξα∂xμdxμ∂ξβ∂xvdxv=gμvdxμdxvdτ2=nαβ∂ξα∂xμdxμ∂ξβ∂xvdxv=gμvdxμdxv
Where gμv=∂ξα∂ξβ∂xμ∂xvηαβgμv=∂ξα∂ξβ∂xμ∂xvηαβ is the metric tensor.
Einstein Tensor
Einstein tensors have no divergence, are symmetric, and they are concomitants with metric tensors gab and its first two derivatives. The metric and Einstein tensors are the only tensors with these properties in four dimensions, and their number depends critically on the dimension of the space.
The Einstein tensor is
Gμv=Rμv−12RgμvGμv=Rμv−12Rgμv
Properties of the Einstein tensor
- When spacetime is flat, it vanishes.
- It is made up of the Riemann and the metric tensor.
- This type of tensor differs from other tensors constructed by using the Riemann tensor and the demand metric.
- It is symmetric and linear in Riemann.
The Cosmological Constant
You may have noticed that a term may be added to Einstein’s field equation which would be consistent with local conservation of TμvTμv. For some constant ΛΛ, this is a term of the form ΛgμvΛgμv. Because the metric’s covariant derivative is zero, adding it to the left side does not affect local conservation. The term is known as the cosmological constant.
Einstein’s initial motivation for introducing ΛΛ was the realization that there were no solutions to his equations representing a static cosmology (a universe that does not change with time on large scales) with a nonzero matter content. Indeed, it was thought at the time that the universe was static. A static solution can be found in the cosmological constant is precisely tuned, but it is unstable to small perturbations.
This changed a few years later when Hubble demonstrated that the universe is expanding and thus not static (as Einstein’s equation would predict if the cosmological constant was not included; Einstein referred to this as the “biggest mistake of his life”). Because of this discovery, Einstein rejected his suggestion.
Practical Application of Einstein Field Equations
- Because of the height of the satellites above the earth, the GPS in your phone relies on relativistic time corrections. (They also require speed corrections, but these can be derived from special relativity without resorting to the field equations.)
- Calculating Mercury’s relativistic precession. This post-diction was a critical factor in the rapid acceptance of general relativity.
- Another example is GPS modeling and calculating the orbits of LAGEOS and Gravity Probe B. Because gravity around extremely massive objects is simple, a full-fledged general relativistic formulation works quite well (and is necessary for) black holes and neutron stars.
Conclusion
Under relativity theory, Einstein’s field equations correspond to spacetime geometrical points. It is determined by the separation of matter within it. Einstein proposed the equation in 1915. It took the shape of a tensor equation by which spacetime curvature is linked to energy, momentum, and stress. Ten equations make up this set which is derived from the General Theory of Relativity of Albert Einstein. In this tutorial, we have described Einstein Field Equations, the metric tensor, the Einstein tensor, the properties of the Einstein tensor, the cosmological constant, practical Application of Einstein Field Equations.
Leave a Reply